| Title Page | Introduction | Ada Lake Info | Boat Info | Calculations | Discussion of Results | Acknowledgements |
Wave Height
Boat Wave Height:
To find the height of the waves generated by the boats, I used the equation summarized by Sorensen and developed by Bhomik, Soong, Reichelt, and Seddik. This equation was produced by measuring the waves produced by 12 different recreation-type vessels. It should be noted that the type of boat hull (V-hull, Tri-hull, Jon boat, etc) was not taken into account.
Hm = 0.537*V-0.346*x-0.345*LV0.56*D0.355
where Hm = maximum wave height in meters, V = velocity in m/s, x = distance from shore in meters, LV = length of vessel in meters
D = Draft of vessel (how deep it sits in the water) in meters
Boat length and draft are shown in the table below.
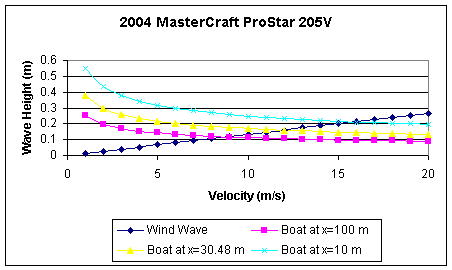
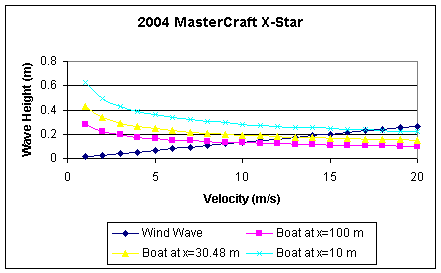
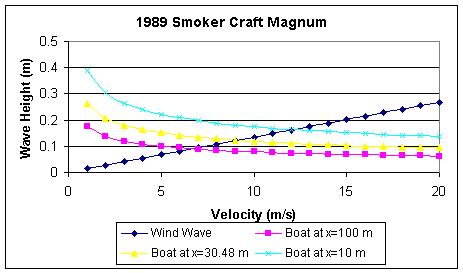
| ProStar 205V | X-Star | Magnum | |
| LV | 6.27 | 6.78 | 4.9 |
| Draft | 0.56 | 0.71 | 0.3048 |
Wind Wave Height:
s for varying wind speed (U) according to the following equations:
Hs/U2 = 0.0016*(Fs/U = 0.286*(F1/3 where F*=gF/U2
The wind was estimated to be coming from the southwest, making the fetch 685.8 m, which is the largest fetch possible on Ada Lake.
For vessel and wind speeds varying from 1 to 20 m/s, and for boat distances from shore varying from 10 to 100 meters, the wave heights are shown below.



The distance of x = 30.48 m corresponds to the distance of 100 ft, which is the closest boats can travel to the shore with a wake in the state of Wisconsin. From the graphs you can see that the wave height observable from shore increases as the boat gets closer to shore. It is noteworthy that the wave heights decrease with an increase in speed, which is a result of the planing effect of boats. Also evident is that the wind wave height increases with wind speed.